1/4 3/8 Reduced To The Lowest Terms
listenit
Apr 05, 2025 · 5 min read
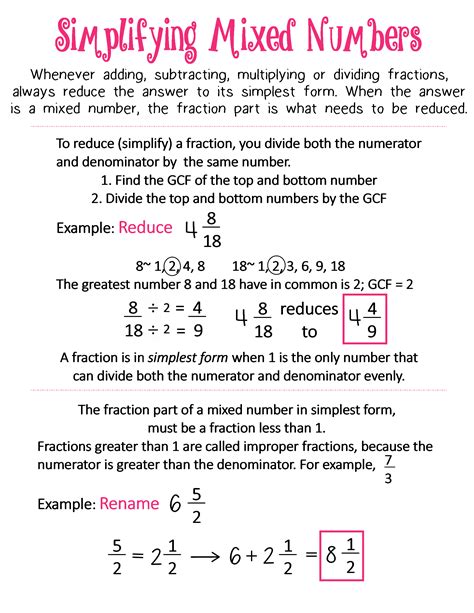
Table of Contents
1/4 and 3/8 Reduced to Lowest Terms: A Deep Dive into Fraction Simplification
Understanding fractions and how to simplify them is a fundamental skill in mathematics. This comprehensive guide will delve into the process of reducing fractions to their lowest terms, using 1/4 and 3/8 as prime examples. We'll explore the underlying concepts, provide step-by-step instructions, and offer numerous examples to solidify your understanding. This isn't just about simplifying fractions; it's about mastering a core mathematical concept with practical applications across various fields.
What are Fractions and Why Simplify Them?
A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). For example, in the fraction 1/4, 1 is the numerator and 4 is the denominator. This represents one out of four equal parts.
Simplifying fractions, also known as reducing fractions to lowest terms, means expressing the fraction in its simplest form. This doesn't change the value of the fraction; it simply makes it easier to understand and work with. A simplified fraction is more concise and avoids unnecessary complexity in calculations.
Why is simplification important?
- Clarity: Simplified fractions are easier to read and understand. Imagine comparing 12/16 and 3/4 – the latter is clearly simpler.
- Efficiency: Simplifying fractions makes calculations faster and less prone to errors. Working with smaller numbers is always more efficient.
- Standardization: In many mathematical contexts, presenting answers in their simplest form is a standard requirement.
- Problem Solving: In real-world applications, a simplified fraction often provides a more intuitive and practical solution.
Finding the Greatest Common Divisor (GCD)
The key to reducing a fraction to its lowest terms is finding the greatest common divisor (GCD), also known as the greatest common factor (GCF), of the numerator and the denominator. The GCD is the largest number that divides both the numerator and the denominator without leaving a remainder.
There are several methods to find the GCD:
1. Listing Factors
This method involves listing all the factors of both the numerator and the denominator and identifying the largest factor they share.
Let's find the GCD of 12 and 16:
- Factors of 12: 1, 2, 3, 4, 6, 12
- Factors of 16: 1, 2, 4, 8, 16
The common factors are 1, 2, and 4. The greatest common factor is 4.
2. Prime Factorization
This method involves expressing both the numerator and the denominator as a product of their prime factors. The GCD is then the product of the common prime factors raised to the lowest power.
Let's find the GCD of 12 and 16 using prime factorization:
- Prime factorization of 12: 2 x 2 x 3 = 2² x 3
- Prime factorization of 16: 2 x 2 x 2 x 2 = 2⁴
The common prime factor is 2, and the lowest power is 2². Therefore, the GCD is 2² = 4.
3. Euclidean Algorithm
This is a more efficient method for larger numbers. It involves repeatedly applying the division algorithm until the remainder is 0. The last non-zero remainder is the GCD.
Let's find the GCD of 12 and 16 using the Euclidean algorithm:
- Divide 16 by 12: 16 = 12 x 1 + 4
- Divide 12 by the remainder 4: 12 = 4 x 3 + 0
The last non-zero remainder is 4, so the GCD is 4.
Reducing 1/4 to Lowest Terms
The fraction 1/4 is already in its lowest terms. This is because the GCD of 1 and 4 is 1. There are no common factors other than 1. Therefore, 1/4 cannot be simplified further.
Reducing 3/8 to Lowest Terms
Similarly, the fraction 3/8 is also in its lowest terms. The GCD of 3 and 8 is 1. 3 is a prime number, and 8 (2 x 2 x 2) doesn't share any prime factors with 3. Therefore, 3/8 cannot be simplified.
Examples of Fraction Simplification
Let's practice with a few more examples:
1. Simplify 12/16:
- Find the GCD of 12 and 16 (which we already determined is 4).
- Divide both the numerator and the denominator by the GCD: 12 ÷ 4 = 3 and 16 ÷ 4 = 4
- The simplified fraction is 3/4.
2. Simplify 15/25:
- Find the GCD of 15 and 25 (which is 5).
- Divide both the numerator and the denominator by 5: 15 ÷ 5 = 3 and 25 ÷ 5 = 5
- The simplified fraction is 3/5.
3. Simplify 24/36:
- Find the GCD of 24 and 36 (which is 12).
- Divide both the numerator and the denominator by 12: 24 ÷ 12 = 2 and 36 ÷ 12 = 3
- The simplified fraction is 2/3.
Real-World Applications of Fraction Simplification
Fraction simplification isn't just an abstract mathematical exercise; it has numerous practical applications in everyday life and various professions:
- Cooking and Baking: Recipes often use fractions to specify ingredient quantities. Simplifying fractions makes it easier to understand and measure ingredients accurately.
- Construction and Engineering: Calculations involving measurements and proportions frequently involve fractions. Simplified fractions enhance accuracy and efficiency in these fields.
- Finance and Accounting: Fractions are used to represent proportions and percentages in financial calculations. Simplification improves the clarity and understanding of financial reports.
- Data Analysis and Statistics: Fractions are commonly used to represent probabilities and proportions in data analysis. Simplified fractions make it easier to interpret results and draw conclusions.
Conclusion: Mastering Fraction Simplification
Reducing fractions to their lowest terms is a crucial skill in mathematics. By understanding the concept of the greatest common divisor and applying the various methods to find it, you can efficiently simplify fractions and improve your mathematical skills. This simplification not only enhances the clarity and efficiency of your work but also provides a foundation for more advanced mathematical concepts. Remember to always express your answers in the simplest form for clarity, accuracy, and standardization. Practice makes perfect, so keep working through examples until you feel confident in your ability to simplify fractions.
Latest Posts
Latest Posts
-
How Many Ounces Are In A Fifth Of Whiskey
Apr 05, 2025
-
Which Element Has Chemical Properties Most Similar To Sodium
Apr 05, 2025
-
What Shape Has 4 Right Angles
Apr 05, 2025
-
Dna Replication Is Said To Be Semiconservative Because
Apr 05, 2025
-
Why Is A Graduated Cylinder More Accurate Than A Beaker
Apr 05, 2025
Related Post
Thank you for visiting our website which covers about 1/4 3/8 Reduced To The Lowest Terms . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
